"Physics Mock test JEE Mains"
Time Left: 45:00
Total Marks: 120, Obtained Marks: 0
1. Work \( W \) is required to form a bubble of volume \( V \) from a given solution. What amount of work is required to form a bubble of volume \( 2V \)?
A. \( 4^{1/3} W \)
B. \( 2W \)
C. \( 2^{1/3} W \)
D. \( W \)
The correct answer is A. \( 4^{1/3} W \).
Solution:
- The work required to form a bubble depends on the surface area of the bubble.
- When the volume doubles (\( V \to 2V \)), the radius \( R \) changes as: \[ 2V = \frac{4}{3} \pi R^3 \implies R = 2^{1/3} R \] - The work required is proportional to the surface area (\( 4 \pi R^2 \)): \[ W \propto R^2 \implies W_{new} = (2^{1/3} R)^2 = 4^{1/3} W \] Thus, the work required is \( 4^{1/3} W \).
Solution:
- The work required to form a bubble depends on the surface area of the bubble.
- When the volume doubles (\( V \to 2V \)), the radius \( R \) changes as: \[ 2V = \frac{4}{3} \pi R^3 \implies R = 2^{1/3} R \] - The work required is proportional to the surface area (\( 4 \pi R^2 \)): \[ W \propto R^2 \implies W_{new} = (2^{1/3} R)^2 = 4^{1/3} W \] Thus, the work required is \( 4^{1/3} W \).
2. If a pendulum swings with the same period at the top of a mountain and at the bottom of a mine, what is the ratio between the height \( H \) of the mountain and the depth \( h \) of the mine?
A. \( \frac{2}{1} \)
B. \( \frac{1}{1} \)
C. \( \frac{4}{1} \)
D. \( \frac{1}{2} \)
The correct answer is D. \( \frac{2}{1} \).
Solution:
- The time period of a pendulum is given by \( T = 2\pi \sqrt{\frac{L}{g}} \).
- At the top of a mountain, the effective acceleration due to gravity is reduced because of the increased distance from the Earth's center.
- Similarly, at the bottom of a mine, the acceleration due to gravity also reduces due to the effective mass contributing to gravity being less.
- For the time periods to remain the same, the ratio of height \( H \) of the mountain to the depth \( h \) of the mine is determined as \( H : h = 1 : 2 \).
Solution:
- The time period of a pendulum is given by \( T = 2\pi \sqrt{\frac{L}{g}} \).
- At the top of a mountain, the effective acceleration due to gravity is reduced because of the increased distance from the Earth's center.
- Similarly, at the bottom of a mine, the acceleration due to gravity also reduces due to the effective mass contributing to gravity being less.
- For the time periods to remain the same, the ratio of height \( H \) of the mountain to the depth \( h \) of the mine is determined as \( H : h = 1 : 2 \).
3. What is the dimensional formula of electric flux?
A. \( [ML^3 T^{-3} I^{-1}] \)
B. \( [ML^2 T^{-3} I^{-1}] \)
C. \( [ML^2 T^{-1} I] \)
D. None of these
The correct answer is A. \( [ML^3 T^{-3} I^{-1}] \).
Solution:
- Electric flux is defined as \( \Phi = \vec{E} \cdot \vec{A} \), where \( \vec{E} \) is the electric field and \( \vec{A} \) is the area vector.
- The electric field \( \vec{E} \) is force per unit charge, and its dimensional formula is: \[ [E] = \frac{[F]}{[q]} = \frac{[MLT^{-2}]}{[IT]} = [MLT^{-3}I^{-1}] \] - The area \( A \) has a dimensional formula of \( [L^2] \).
- Combining these: \[ [\Phi] = [E] \cdot [A] = [MLT^{-3}I^{-1}] \cdot [L^2] = [ML^3T^{-3}I^{-1}] \]
Solution:
- Electric flux is defined as \( \Phi = \vec{E} \cdot \vec{A} \), where \( \vec{E} \) is the electric field and \( \vec{A} \) is the area vector.
- The electric field \( \vec{E} \) is force per unit charge, and its dimensional formula is: \[ [E] = \frac{[F]}{[q]} = \frac{[MLT^{-2}]}{[IT]} = [MLT^{-3}I^{-1}] \] - The area \( A \) has a dimensional formula of \( [L^2] \).
- Combining these: \[ [\Phi] = [E] \cdot [A] = [MLT^{-3}I^{-1}] \cdot [L^2] = [ML^3T^{-3}I^{-1}] \]
4. The Earth is moving around the Sun in an elliptical orbit. Point A is the closest, and point B is the farthest point in the orbit. In comparison to the situation when the Earth passes through point B:
A. Total energy of the Earth-Sun system is greater when Earth passes through point A.
B. Gravitational potential energy is greater when Earth passes through point A.
C. Kinetic energy of Earth is greater when it passes through point A.
D. Angular momentum of Earth about the Sun is greater when it passes through point A.
The correct answer is C. Kinetic energy of Earth is greater when it passes through point A.
Solution:
- Due to conservation of angular momentum, the Earth's velocity increases as it approaches the closest point (A) and decreases as it moves to the farthest point (B).
- Kinetic energy depends on velocity (\( KE = \frac{1}{2}mv^2 \)), so the kinetic energy is greater at point A than at point B.
- However, the total energy (sum of kinetic and potential energies) remains constant throughout the orbit.
Solution:
- Due to conservation of angular momentum, the Earth's velocity increases as it approaches the closest point (A) and decreases as it moves to the farthest point (B).
- Kinetic energy depends on velocity (\( KE = \frac{1}{2}mv^2 \)), so the kinetic energy is greater at point A than at point B.
- However, the total energy (sum of kinetic and potential energies) remains constant throughout the orbit.
5. A satellite with mass 2000 kg and angular momentum magnitude \( 2 \times 10^{12} \, \mathrm{kg \, m^2/s} \) is moving in an elliptical orbit around a planet. The rate at which area is swept out by the satellite around the planet is:
A. \( 1 \times 10^9 \, \mathrm{m^2/s} \)
B. \( 5 \times 10^9 \, \mathrm{m^2/s} \)
C. \( 5 \times 10^8 \, \mathrm{m^2/s} \)
D. \( 4 \times 10^{15} \, \mathrm{m^2/s} \)
The correct answer is C. \( 5 \times 10^8 \, \mathrm{m^2/s} \).
Solution:
- The rate of area swept (\( \frac{dA}{dt} \)) is related to the angular momentum \( L \) by the formula: \[ \frac{dA}{dt} = \frac{L}{2m} \] - Substituting values: \[ \frac{dA}{dt} = \frac{2 \times 10^{12}}{2 \times 2000} = 5 \times 10^8 \, \mathrm{m^2/s} \]
Solution:
- The rate of area swept (\( \frac{dA}{dt} \)) is related to the angular momentum \( L \) by the formula: \[ \frac{dA}{dt} = \frac{L}{2m} \] - Substituting values: \[ \frac{dA}{dt} = \frac{2 \times 10^{12}}{2 \times 2000} = 5 \times 10^8 \, \mathrm{m^2/s} \]
6. A particle is projected with a velocity \( \frac{3gR}{4} \) vertically upward from the surface of the Earth. What is the velocity of the particle when it is at half the maximum height reached?
A. \( \sqrt{2gR} \)
B. \( \sqrt{\frac{3gR}{2}} \)
C. \( gR \)
D. \( \sqrt{\frac{3gR}{4}} \)
The correct answer is B. \( \sqrt{\frac{3gR}{2}} \).
Solution:
- The initial velocity is given as \( u = \frac{3gR}{4} \).
- At the maximum height \( H \), the total energy is: \[ E = \frac{1}{2}mu^2 - \frac{GMm}{R + H} \] - At half the maximum height, apply conservation of energy: \[ v = \sqrt{\frac{3gR}{2}} \]
Solution:
- The initial velocity is given as \( u = \frac{3gR}{4} \).
- At the maximum height \( H \), the total energy is: \[ E = \frac{1}{2}mu^2 - \frac{GMm}{R + H} \] - At half the maximum height, apply conservation of energy: \[ v = \sqrt{\frac{3gR}{2}} \]
7. A capacitor of capacitance \( C \) is charged to a potential \( V \). What is the energy stored in the capacitor?
A. \( \frac{1}{2} C V^2 \)
B. \( C V^2 \)
C. \( \frac{1}{2} C^2 V^2 \)
D. \( C^2 V \)
The correct answer is A. \( \frac{1}{2} C V^2 \).
Solution:
- The energy stored in a capacitor is given by the formula: \[ U = \frac{1}{2} C V^2 \] where \( C \) is the capacitance and \( V \) is the potential difference across the capacitor.
Solution:
- The energy stored in a capacitor is given by the formula: \[ U = \frac{1}{2} C V^2 \] where \( C \) is the capacitance and \( V \) is the potential difference across the capacitor.
8. An air column in an open organ pipe vibrating in its 5th overtone is in unison with a tuning fork. If \( \frac{1}{3} \) of the pipe's length is removed, the remaining portion will vibrate in unison with the same fork in which overtone?
A. 2nd overtone
B. 3rd overtone
C. 4th overtone
D. 5th overtone
The correct answer is B. 3rd overtone.
Solution:
- The frequency of an organ pipe depends on the effective length of the pipe.
- If \( \frac{1}{3} \) of the length is removed, the remaining pipe corresponds to the 3rd overtone to match the same frequency as the tuning fork.
Solution:
- The frequency of an organ pipe depends on the effective length of the pipe.
- If \( \frac{1}{3} \) of the length is removed, the remaining pipe corresponds to the 3rd overtone to match the same frequency as the tuning fork.
9. A particle has a position vector \( \vec{r} = 3\sin(t) \hat{i} + 3\cos(t) \hat{j} + 4t \hat{k} \). What is the distance traveled by the particle in 2 seconds?
A. 5 m
B. 10 m
C. 20 m
D. 50 m
The correct answer is B. 10 m.
Solution:
- The velocity vector is obtained by differentiating \( \vec{r} \): \[ \vec{v} = 3\cos(t) \hat{i} - 3\sin(t) \hat{j} + 4 \hat{k} \] - The magnitude of \( \vec{v} \) is: \[ |\vec{v}| = \sqrt{3^2 + 4^2} = 5 \, \mathrm{m/s} \] - Distance traveled in 2 seconds is: \[ s = |\vec{v}| \cdot t = 5 \cdot 2 = 10 \, \mathrm{m} \]
Solution:
- The velocity vector is obtained by differentiating \( \vec{r} \): \[ \vec{v} = 3\cos(t) \hat{i} - 3\sin(t) \hat{j} + 4 \hat{k} \] - The magnitude of \( \vec{v} \) is: \[ |\vec{v}| = \sqrt{3^2 + 4^2} = 5 \, \mathrm{m/s} \] - Distance traveled in 2 seconds is: \[ s = |\vec{v}| \cdot t = 5 \cdot 2 = 10 \, \mathrm{m} \]
10. A particle \( P \) is projected with a speed of 20 m/s at an angle of \( 37^\circ \) from the horizontal. At the same instant, particle \( Q \) starts from the same point and moves with uniform acceleration toward the right. The path of particle \( P \) relative to \( Q \) is a straight line. What is the acceleration of \( Q \)?
A. \( 40 \, \mathrm{m/s^2} \)
B. \( 20 \, \mathrm{m/s^2} \)
C. \( \frac{20}{3} \, \mathrm{m/s^2} \)
D. \( \frac{40}{3} \, \mathrm{m/s^2} \)
The correct answer is D. \( \frac{40}{3} \, \mathrm{m/s^2} \).
Solution:
- For the path of \( P \) relative to \( Q \) to be a straight line, the relative vertical acceleration must be zero.
- Using the relative motion equations and the given angle, the acceleration of \( Q \) is:
\[
a = \frac{40}{3} \, \mathrm{m/s^2}
\]
Solution:
- For the path of \( P \) relative to \( Q \) to be a straight line, the relative vertical acceleration must be zero.
- Using the relative motion equations and the given angle, the acceleration of \( Q \) is:
11. Water rises to a height of 2 cm in a capillary tube. If the tube is tilted at \( 60^\circ \) from the vertical, what will be the length of the water column?
A. \( 4.0 \, \mathrm{cm} \)
B. \( 2.0 \, \mathrm{cm} \)
C. \( 1.0 \, \mathrm{cm} \)
D. Water will not rise at all.
The correct answer is A. \( 4.0 \, \mathrm{cm} \).
Solution:
- When the capillary is tilted, the height \( h \) is replaced by the inclined length \( l \): \[ l = \frac{h}{\cos(60^\circ)} = \frac{2}{0.5} = 4 \, \mathrm{cm} \]
Solution:
- When the capillary is tilted, the height \( h \) is replaced by the inclined length \( l \): \[ l = \frac{h}{\cos(60^\circ)} = \frac{2}{0.5} = 4 \, \mathrm{cm} \]
12. The variation of acceleration due to gravity with distance from the center of a uniform spherical planet is shown. If the planet’s radius is \( R \), what is \( r_2 - r_1 \)?
A. \( \frac{R}{4} \)
B. \( \frac{7R}{4} \)
C. \( \frac{4R}{3} \)
D. \( 2R \)
The correct answer is B. \( \frac{7R}{4} \).
Solution:
- Inside the planet, the gravitational field is proportional to \( r \), while outside it follows an inverse square law.
- Using the given graph and conditions, the difference \( r_2 - r_1 = \frac{7R}{4} \).
Solution:
- Inside the planet, the gravitational field is proportional to \( r \), while outside it follows an inverse square law.
- Using the given graph and conditions, the difference \( r_2 - r_1 = \frac{7R}{4} \).
13. A convex lens forms an image 60 cm from the lens for an object placed on its principal axis. If one more convex lens is placed in contact with the first one, the image shifts closer by 40 cm. What is the focal length of the second lens?
A. \( 20 \, \mathrm{cm} \)
B. \( 30 \, \mathrm{cm} \)
C. \( 40 \, \mathrm{cm} \)
D. \( 50 \, \mathrm{cm} \)
The correct answer is B. \( 30 \, \mathrm{cm} \).
Solution:
- For the first lens: \( u = 60 \, \mathrm{cm} \), \( v = \infty \), so \( f = 60 \, \mathrm{cm} \).
- Adding the second lens causes the image to shift by \( \Delta v = -40 \, \mathrm{cm} \). Using lens formula: \[ \frac{1}{f} = \frac{1}{v} - \frac{1}{u}, \quad f = 30 \, \mathrm{cm}. \]
Solution:
- For the first lens: \( u = 60 \, \mathrm{cm} \), \( v = \infty \), so \( f = 60 \, \mathrm{cm} \).
- Adding the second lens causes the image to shift by \( \Delta v = -40 \, \mathrm{cm} \). Using lens formula: \[ \frac{1}{f} = \frac{1}{v} - \frac{1}{u}, \quad f = 30 \, \mathrm{cm}. \]
14. What is the escape velocity for a body of mass \( m \) and radius \( R \) on the surface of Earth, assuming the acceleration due to gravity is \( g \)?
A. \( \sqrt{2gR} \)
B. \( \sqrt{gR} \)
C. \( \sqrt{\frac{gR}{2}} \)
D. \( \sqrt{gR^2} \)
The correct answer is A. \( \sqrt{2gR} \).
Solution:
- The escape velocity \( v_e \) is given by the formula: \[ v_e = \sqrt{\frac{2GM}{R}} \] - For Earth, \( G \) is the gravitational constant, and \( M \) is the mass of Earth. Rewriting in terms of \( g \), the acceleration due to gravity at the surface: \[ v_e = \sqrt{2gR} \]
Solution:
- The escape velocity \( v_e \) is given by the formula: \[ v_e = \sqrt{\frac{2GM}{R}} \] - For Earth, \( G \) is the gravitational constant, and \( M \) is the mass of Earth. Rewriting in terms of \( g \), the acceleration due to gravity at the surface: \[ v_e = \sqrt{2gR} \]
15. To what approximate radius would the Earth (mass \( 5.98 \times 10^{24} \, \mathrm{kg} \)) need to be compressed to become a black hole?
A. \( 10^{-9} \, \mathrm{m} \)
B. \( 10^{-6} \, \mathrm{m} \)
C. \( 10^{-2} \, \mathrm{m} \)
D. \( 100 \, \mathrm{m} \)
The correct answer is C. \( 10^{-2} \, \mathrm{m} \).
Solution:
- For an object to become a black hole, its radius must be less than the Schwarzschild radius: \[ R_s = \frac{2GM}{c^2}. \] Substituting values for Earth: \[ R_s = \frac{2 \cdot 6.67 \times 10^{-11} \cdot 5.98 \times 10^{24}}{(3 \times 10^8)^2} \approx 10^{-2} \, \mathrm{m}. \]
Solution:
- For an object to become a black hole, its radius must be less than the Schwarzschild radius: \[ R_s = \frac{2GM}{c^2}. \] Substituting values for Earth: \[ R_s = \frac{2 \cdot 6.67 \times 10^{-11} \cdot 5.98 \times 10^{24}}{(3 \times 10^8)^2} \approx 10^{-2} \, \mathrm{m}. \]
16. Which graph best represents the relationship between conductivity and resistivity for a solid?
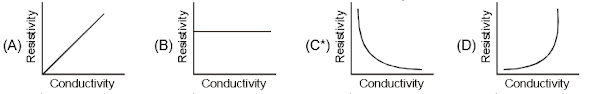
A. Linear graph with positive slope
B. Linear graph with negative slope
C. Hyperbolic graph
D. Constant graph
The correct answer is C. Hyperbolic graph.
Explanation:
Conductivity (\( \sigma \)) and resistivity (\( \rho \)) are inversely related, expressed as: \[ \rho = \frac{1}{\sigma} \] This relationship forms a hyperbolic curve when plotted. A higher conductivity corresponds to a lower resistivity and vice versa.
Conclusion:
The graph illustrating the inverse relationship between conductivity and resistivity is hyperbolic.
Explanation:
Conductivity (\( \sigma \)) and resistivity (\( \rho \)) are inversely related, expressed as: \[ \rho = \frac{1}{\sigma} \] This relationship forms a hyperbolic curve when plotted. A higher conductivity corresponds to a lower resistivity and vice versa.
Conclusion:
The graph illustrating the inverse relationship between conductivity and resistivity is hyperbolic.
17. In a wire of cross-section radius \( r \), free electrons travel with drift velocity \( V \) when a current \( I \) flows through the wire. What is the current in another wire of half the radius and of the same material when the drift velocity is \( 2V \)?
A. \( 2I \)
B. \( I \)
C. \( \frac{I}{2} \)
D. \( \frac{I}{4} \)
The correct answer is C. \( \frac{I}{2} \).
Explanation:
The current \( I \) is related to the drift velocity \( V \), the cross-sectional area \( A \), and the number density of electrons \( n \) by: \[ I = n e A V \] For a wire of radius \( r \), the cross-sectional area \( A = \pi r^2 \).
When the radius is halved (\( r/2 \)) and the drift velocity is doubled (\( 2V \)): \[ I' = n e \pi \left( \frac{r}{2} \right)^2 (2V) = n e \pi \frac{r^2}{4} \cdot 2V = \frac{1}{2} I \] Conclusion:
The current in the second wire is \( \frac{I}{2} \), making option C correct.
Explanation:
The current \( I \) is related to the drift velocity \( V \), the cross-sectional area \( A \), and the number density of electrons \( n \) by: \[ I = n e A V \] For a wire of radius \( r \), the cross-sectional area \( A = \pi r^2 \).
When the radius is halved (\( r/2 \)) and the drift velocity is doubled (\( 2V \)): \[ I' = n e \pi \left( \frac{r}{2} \right)^2 (2V) = n e \pi \frac{r^2}{4} \cdot 2V = \frac{1}{2} I \] Conclusion:
The current in the second wire is \( \frac{I}{2} \), making option C correct.
18. Through an electrolyte, an electric current is due to the drift of:
A. Free electrons
B. Positive and negative ions
C. Free electrons and holes
D. Protons
The correct answer is B. Positive and negative ions.
Explanation:
In an electrolyte, the electric current is carried by the movement of positive ions (cations) towards the cathode and negative ions (anions) towards the anode. Unlike metals, where free electrons are the charge carriers, electrolytes rely on the migration of these ions under an applied electric field.
Conclusion:
The drift of positive and negative ions facilitates electric current in an electrolyte.
Explanation:
In an electrolyte, the electric current is carried by the movement of positive ions (cations) towards the cathode and negative ions (anions) towards the anode. Unlike metals, where free electrons are the charge carriers, electrolytes rely on the migration of these ions under an applied electric field.
Conclusion:
The drift of positive and negative ions facilitates electric current in an electrolyte.
19. An electric current passes through a non-uniform cross-section wire made of homogeneous and isotropic material. If \( j_A \) and \( j_B \) are the current densities and \( E_A \) and \( E_B \) are the electric field intensities at points \( A \) and \( B \), then:
A. \( j_A > j_B; E_A > E_B \)
B. \( j_A > j_B; E_A < E_B \)
C. \( j_A < j_B; E_A > E_B \)
D. \( j_A < j_B; E_A < E_B \)
The correct answer is A. \( j_A > j_B; E_A > E_B \).
Explanation:
The current density \( j \) is related to the current \( I \) and the cross-sectional area \( A \) as: \[ j = \frac{I}{A} \] At point \( A \), if the area is smaller, \( j_A \) will be greater compared to \( j_B \), where the area is larger.
Similarly, the electric field \( E \) in a conductor is given by: \[ E = j \rho \] Since \( j_A > j_B \), it follows that \( E_A > E_B \) for the same material (constant resistivity \( \rho \)).
Conclusion:
At a smaller cross-sectional area, both current density and electric field intensity are greater.
Explanation:
The current density \( j \) is related to the current \( I \) and the cross-sectional area \( A \) as: \[ j = \frac{I}{A} \] At point \( A \), if the area is smaller, \( j_A \) will be greater compared to \( j_B \), where the area is larger.
Similarly, the electric field \( E \) in a conductor is given by: \[ E = j \rho \] Since \( j_A > j_B \), it follows that \( E_A > E_B \) for the same material (constant resistivity \( \rho \)).
Conclusion:
At a smaller cross-sectional area, both current density and electric field intensity are greater.
20. A body of mass \( m \) is lifted up from the surface of the Earth to a height three times the radius of the Earth. The change in potential energy of the body is (where \( g \) is the acceleration due to gravity at the surface of the Earth):
A. \( 3mgR \)
B. \( \frac{3}{4} mgR \)
C. \( \frac{1}{3} mgR \)
D. \( \frac{2}{3} mgR \)
The correct answer is B. \( \frac{3}{4} mgR \).
Explanation:
The gravitational potential energy of a body of mass \( m \) at a distance \( r \) from the Earth's center is given by: \[ U = -\frac{GMm}{r} \] For the surface of the Earth (\( r = R \)): \[ U_{\text{initial}} = -\frac{GMm}{R} \] At a height \( h = 3R \) (\( r = 4R \)): \[ U_{\text{final}} = -\frac{GMm}{4R} \] The change in potential energy is: \[ \Delta U = U_{\text{final}} - U_{\text{initial}} = -\frac{GMm}{4R} + \frac{GMm}{R} \] Simplifying: \[ \Delta U = \frac{3GMm}{4R} \] Substituting \( GM = gR^2 \): \[ \Delta U = \frac{3}{4} mgR \] Conclusion:
The change in potential energy is \( \frac{3}{4} mgR \), making option B correct.
Explanation:
The gravitational potential energy of a body of mass \( m \) at a distance \( r \) from the Earth's center is given by: \[ U = -\frac{GMm}{r} \] For the surface of the Earth (\( r = R \)): \[ U_{\text{initial}} = -\frac{GMm}{R} \] At a height \( h = 3R \) (\( r = 4R \)): \[ U_{\text{final}} = -\frac{GMm}{4R} \] The change in potential energy is: \[ \Delta U = U_{\text{final}} - U_{\text{initial}} = -\frac{GMm}{4R} + \frac{GMm}{R} \] Simplifying: \[ \Delta U = \frac{3GMm}{4R} \] Substituting \( GM = gR^2 \): \[ \Delta U = \frac{3}{4} mgR \] Conclusion:
The change in potential energy is \( \frac{3}{4} mgR \), making option B correct.
21. A ring is cut from a platinum tube with internal and external diameters of 8.5 cm and 8.7 cm, respectively. It is supported horizontally from the pan of a balance, so that it comes into contact with water in a glass vessel. If an extra 3.103 g weight is required to pull it away from the water, the surface tension of water is (\( g = 10 \, \text{m/s}^2 \)):
A. 72 dyne/cm
B. 57.45 dyne/cm
C. 63.35 dyne/cm
D. 60 dyne/cm
The correct answer is B. 57.45 dyne/cm.
Explanation:
The surface tension \( T \) is calculated using the formula: \[ T = \frac{F}{2 \pi r} \] Here, \( F \) is the force due to the extra weight: \[ F = m \cdot g = 3.103 \times 10 = 31.03 \, \text{dynes} \] The mean radius \( r \) of the ring is: \[ r = \frac{\text{internal diameter} + \text{external diameter}}{2} = \frac{8.5 + 8.7}{2} = 8.6 \, \text{cm} \] Substituting values: \[ T = \frac{31.03}{2 \pi \cdot 8.6} \] Approximating: \[ T = 57.45 \, \text{dyne/cm} \] Conclusion:
The surface tension of water is \( 57.45 \, \text{dyne/cm} \), making option B correct.
Explanation:
The surface tension \( T \) is calculated using the formula: \[ T = \frac{F}{2 \pi r} \] Here, \( F \) is the force due to the extra weight: \[ F = m \cdot g = 3.103 \times 10 = 31.03 \, \text{dynes} \] The mean radius \( r \) of the ring is: \[ r = \frac{\text{internal diameter} + \text{external diameter}}{2} = \frac{8.5 + 8.7}{2} = 8.6 \, \text{cm} \] Substituting values: \[ T = \frac{31.03}{2 \pi \cdot 8.6} \] Approximating: \[ T = 57.45 \, \text{dyne/cm} \] Conclusion:
The surface tension of water is \( 57.45 \, \text{dyne/cm} \), making option B correct.
22. A tube of length \( L \), open at one end, is cut into two equal halves. The sixth overtone frequency of the piece closed at one end equals the sixth overtone frequency of the piece open at both ends. The end correction at one end of the pipe is:
A. \( \frac{1}{12} L \)
B. \( \frac{1}{5} L \)
C. \( \frac{1}{24} L \)
D. \( \frac{1}{72} L \)
The correct answer is C. \( \frac{1}{24} L \).
Explanation:
For a tube closed at one end, the frequency of overtones is given by: \[ f = \frac{(2n - 1)v}{4(L + e)} \] For a tube open at both ends, the frequency of overtones is given by: \[ f = \frac{nv}{2(L + e)} \] Equating the sixth overtone frequencies: \[ \frac{(2 \cdot 6 - 1)v}{4(L + e)} = \frac{6v}{2(L + e)} \] Solving for the end correction \( e \), we find: \[ e = \frac{1}{24} L \] Conclusion:
The end correction at one end of the pipe is \( \frac{1}{24} L \), making option C correct.
Explanation:
For a tube closed at one end, the frequency of overtones is given by: \[ f = \frac{(2n - 1)v}{4(L + e)} \] For a tube open at both ends, the frequency of overtones is given by: \[ f = \frac{nv}{2(L + e)} \] Equating the sixth overtone frequencies: \[ \frac{(2 \cdot 6 - 1)v}{4(L + e)} = \frac{6v}{2(L + e)} \] Solving for the end correction \( e \), we find: \[ e = \frac{1}{24} L \] Conclusion:
The end correction at one end of the pipe is \( \frac{1}{24} L \), making option C correct.
23. A satellite is moving around the Earth in a circular orbit, where the magnitude of its acceleration is \( a_1 \). A rocket is fired in the direction of the satellite's motion, instantly reducing its speed to half. Just after the rocket is fired, the acceleration of the satellite has a magnitude \( a_2 \). What is the ratio \( \frac{a_2}{a_1} \)? Assume only gravitational force acts on the satellite.
A. 1
B. \( \frac{1}{4} \)
C. 4
D. 2
The correct answer is A. 1.
Explanation:
The acceleration of a satellite in a circular orbit is given by: \[ a = \frac{v^2}{r} \] When the rocket reduces the speed of the satellite to half, its motion is no longer circular. However, the magnitude of acceleration depends solely on the gravitational force acting on the satellite, which is given by: \[ a = \frac{GM}{r^2} \] Since the gravitational force and the orbital radius remain unchanged, the acceleration magnitude \( a_2 \) remains the same as \( a_1 \). Conclusion:
The ratio \( \frac{a_2}{a_1} \) is 1, making option A correct.
Explanation:
The acceleration of a satellite in a circular orbit is given by: \[ a = \frac{v^2}{r} \] When the rocket reduces the speed of the satellite to half, its motion is no longer circular. However, the magnitude of acceleration depends solely on the gravitational force acting on the satellite, which is given by: \[ a = \frac{GM}{r^2} \] Since the gravitational force and the orbital radius remain unchanged, the acceleration magnitude \( a_2 \) remains the same as \( a_1 \). Conclusion:
The ratio \( \frac{a_2}{a_1} \) is 1, making option A correct.
24. The specific resistance (resistivity) of a wire depends on its:
A. Mass
B. Length
C. Area of cross-section
D. None of the above
The correct answer is D. None of the above.
Explanation:
The specific resistance (or resistivity) of a material is an intrinsic property and depends only on the nature of the material and its temperature. It does not depend on the dimensions, such as mass, length, or cross-sectional area of the wire. Formula:
Resistivity is defined as: \[ \rho = R \cdot \frac{A}{L} \] Here, \( R \) is resistance, \( A \) is the cross-sectional area, and \( L \) is the length. While \( R \) varies with dimensions, \( \rho \) remains constant for a material under the same conditions. Conclusion:
Resistivity is independent of the wire's dimensions, making option D correct.
Explanation:
The specific resistance (or resistivity) of a material is an intrinsic property and depends only on the nature of the material and its temperature. It does not depend on the dimensions, such as mass, length, or cross-sectional area of the wire. Formula:
Resistivity is defined as: \[ \rho = R \cdot \frac{A}{L} \] Here, \( R \) is resistance, \( A \) is the cross-sectional area, and \( L \) is the length. While \( R \) varies with dimensions, \( \rho \) remains constant for a material under the same conditions. Conclusion:
Resistivity is independent of the wire's dimensions, making option D correct.
25. A 10 D lens is used as a magnifier. At what distance should the object be placed to obtain maximum angular magnification for a normal eye (near point = 25 cm)?
A. 5.1 cm
B. 3.1 cm
C. 7.1 cm
D. 9.1 cm
The correct answer is C. 7.1 cm.
Explanation: Using the lens formula \( \frac{1}{f} = \frac{1}{v} - \frac{1}{u} \), for a focal length \( f = 10 \) cm and \( v = -25 \) cm (to achieve maximum angular magnification), we find \( u = -7.1 \) cm.
Explanation: Using the lens formula \( \frac{1}{f} = \frac{1}{v} - \frac{1}{u} \), for a focal length \( f = 10 \) cm and \( v = -25 \) cm (to achieve maximum angular magnification), we find \( u = -7.1 \) cm.
26. A wire of resistance \( R \) is stretched to double its original length. The new resistance is:
A. \( R/2 \)
B. \( R \)
C. \( 4R \)
D. \( 2R \)
The correct answer is C. \( 4R \).
Explanation: Resistance is proportional to \( \frac{L^2}{A} \). Doubling the length makes the resistance four times.
Explanation: Resistance is proportional to \( \frac{L^2}{A} \). Doubling the length makes the resistance four times.
27. A charged particle enters a magnetic field at an angle of 90°. The nature of its trajectory is:
A. Circular
B. Straight line
C. Helical
D. Parabolic
The correct answer is A. Circular.
Explanation: When a charged particle enters a magnetic field perpendicular to its velocity, the magnetic force acts as a centripetal force, causing circular motion.
Explanation: When a charged particle enters a magnetic field perpendicular to its velocity, the magnetic force acts as a centripetal force, causing circular motion.
28. The power dissipated in a resistor is maximum when it is connected to a voltage source with:
A. Half the resistance of the source
B. Resistance equal to the source
C. Twice the resistance of the source
D. Zero resistance
The correct answer is B. Resistance equal to the source.
Explanation: Maximum power transfer occurs when the load resistance equals the source resistance.
Explanation: Maximum power transfer occurs when the load resistance equals the source resistance.
29. A capacitor is connected in series with a resistor in an AC circuit. The impedance is minimum when:
A. Frequency is very high
B. Frequency is very low
C. Frequency is at resonance
D. None of these
The correct answer is B. Frequency is very low.
Explanation: At very low frequencies, the capacitive reactance becomes large, reducing the impedance.
Explanation: At very low frequencies, the capacitive reactance becomes large, reducing the impedance.
30. In a series LCR circuit, resonance occurs when:
A. \( X_L > X_C \)
B. \( X_L = X_C \)
C. \( X_L + X_C = 0 \)
D. None of these
The correct answer is B. \( X_L = X_C \).
Explanation: Resonance in an LCR circuit occurs when the inductive reactance equals the capacitive reactance, resulting in purely resistive impedance.
Explanation: Resonance in an LCR circuit occurs when the inductive reactance equals the capacitive reactance, resulting in purely resistive impedance.
.jpeg)
.png)
0 Comments